One section in the auditorium has 59 rows
of seats. There are 38 seats in each row.
How many seats are in that section?
Answers
Because if there are 59 rows of seats in that section and 38 seats in each row you would do 59x38
Related Questions
A building across the street casts a shadow that is 24 feet long.
Matt is 6 feet tall and casts a shadow that is 4 feet long.
The two triangles formed are similar because the angle to the sun is the same.
What is the height of the building?
Answers
Answer:
The building is 36 feet tall.
Step-by-step explanation:
x/6 = 24/4
4x=144
4x/4 = 144/4
x=36
by how much is the product of 9/5 and 8 1/4 greater than 5
Answers
Answer:
1
Step-by-step explanation:
Just break it into a decimal.. divide the top by bottom than subtract 5
9/4.... 9 divided by 4 is 2.25-5= 2.75
8 1/4...1 divided by 4 is .25 add 8
8.25-5= 3.25
Now add 3.25+2.75=6
So 6 is greater than 5 by 1
Your answer is 1
Guys pls help me!!!!!

Answers
Answer:
13 1/5 Kilometers
Step-by-step explanation:
you times 4 1/2 by 3 because if she runs 4 1/2 in a third of an hour then she runs 4 1/2 3 times every hour.
in linear programming, a solution that does not simultaneously satisfy all constraints is called an part 2 a. intermediate solution. b. impossible solution. c. infeasible solution. d. illogical solution.
Answers
A solution that does not simultaneously satisfy all constraints is called an infeasible solution.
Option (C) is correct.
What is linear programming?
Linear programming, also known as linear optimization, is a method for achieving the best result in a mathematical model with requirements represented by linear relationships. Linear programming is a special case of mathematical programming.
In linear programming, a solution that does not simultaneously satisfy all constraints is called an infeasible solution.
In some cases, there is no feasible solution area, i.e., there are no points that satisfy all constraints of the problem. An infeasible LP problem with two decision variables can be identified through its graph. For example, let us consider the following linear programming problem.
Minimize z = 200x1 + 300x2
subject to
2x1 + 3x2 ≥ 1200
x1 + x2 ≤ 400
2x1 + 1.5x2 ≥ 900
x1, x2 ≥ 0
The region located on the right of PQR includes all solutions, which satisfy the first and the third constraints. The region located on the left of ST includes all solutions, which satisfy the second constraint. Thus, the problem is infeasible because there is no set of points that satisfies all three constraints.
Hence, a solution that does not simultaneously satisfy all constraints is called an infeasible solution.
To learn more about linear programming, visit:
https://brainly.com/question/25828237
#SPJ4

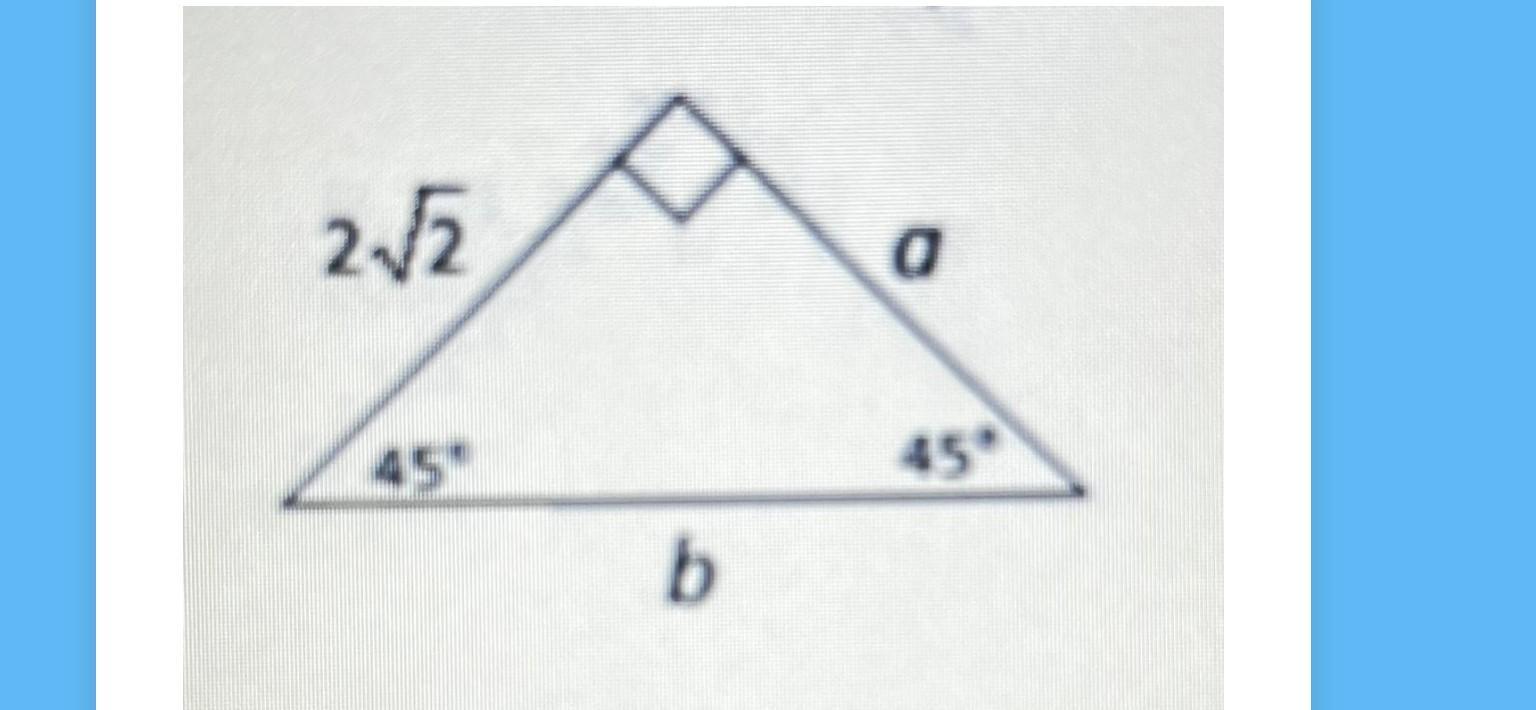
Special right triangle

Answers
Using properties of right angle, The value of a will be 32 units.
What is the special right triangle rule?Particular Right Triangle Formula
The right triangle formula, which states that the square of a right-angled triangle's right triangle is equal to the product of its other two squares, is the fundamental Pythagoras theorem formula.
How long are the other two legs if a hypotenuse of a triangle with a 45°, 45°, and 90° angle is 32 units.
We are aware that Leg: Ankle: Length x = x: x: x2 is the formula for a triangle with a 45°, 45°, and 90° angle.
We can state that x2 = 32 because it is a known that the hypotenuse equals 32. As a result, x = 3 is determined.
After replacing this number of x = 3, the lengths of the empty sides may now be determined.
To know more about right triangle visit:
brainly.com/question/4364353
#SPJ1
Complete question -
Find values of a and b -
two times the sum of a number and 7
Answers
Answer:
2(x+7)
Step-by-step explanation:
i hope this helps
If a two sided test of hypothesis is conducted at a 0.05 level of significance and the test statistic resulting from the analysis was 1.23 . The potential type of statistical error is : No error Type I error Type II error Question 11 1 pts An educational researcher claims that the mean GPA for Psychology students at a certain college is less than 3.2 . A sample of 49 Psychology students gave a mean GPA of 3.1 with a standard deviation 0.35 . What is the value of the test statistic used to test the claim ? ( Do not round) Question 12 1 pts An educational researcher claims that the mean GPA for Psychology students at a certain college is equal to 3.2 . To test this claim a sample of 49 randomly selected Psychology students was selected . The mean GPA was 3.1 with a standard deviation 0.35 . What is the p-value of the test ? ( Round to three decimal places )
Answers
The value of the test statistic used to test the claim is -2.00.
And, at a significance level of 0.05, we fail to reject the null hypothesis and conclude that we do not have sufficient evidence to support the claim that the mean GPA for Psychology students at the college is equal to 3.2.
Now, If a two-sided test of hypothesis is conducted at a 0.05 level of significance and the test statistic resulting from the analysis was 1.23, the potential type of statistical error is Type II error.
A Type II error occurs when we fail to reject a false null hypothesis, meaning that we conclude there is no significant difference or effect when there actually is one.
To answer the second question, we can perform a one-sample t-test to test the claim that the mean GPA for Psychology students at a certain college is less than 3.2.
The hypotheses are:
H₀: μ = 3.2
Ha: μ < 3.2
where μ is the population mean GPA.
We can use the t-statistic formula to calculate the test statistic:
t = (x - μ) / (s / √n)
where, x is the sample mean GPA, s is the sample standard deviation, n is the sample size, and μ is the hypothesized population mean.
Substituting the given values, we get:
t = (3.1 - 3.2) / (0.35 / √49)
t = -0.10 / 0.05
t = -2.00
Therefore, the value of the test statistic used to test the claim is -2.00.
Since this is a one-tailed test with a significance level of 0.05, we compare the t-statistic to the critical t-value from a t-table with 48 degrees of freedom.
At a significance level of 0.05 and 48 degrees of freedom, the critical t-value is -1.677.
Since the calculated t-statistic (-2.00) is less than the critical t-value (-1.677), we reject the null hypothesis and conclude that there is sufficient evidence to support the claim that the mean GPA for Psychology students at the college is less than 3.2.
To calculate the p-value of the test, we can perform a one-sample t-test using the formula:
t = (x - μ) / (s / √n)
where x is the sample mean GPA, μ is the hypothesized population mean GPA, s is the sample standard deviation, and n is the sample size.
Substituting the given values, we get:
t = (3.1 - 3.2) / (0.35 / √49)
t = -0.10 / 0.05
t = -2.00
The degrees of freedom for this test is 49 - 1 = 48.
Using a t-distribution table or calculator, we can find the probability of getting a t-value as extreme as -2.00 or more extreme under the null hypothesis.
Since this is a two-sided test, we need to find the area in both tails beyond |t| = 2.00. The p-value is the sum of these two areas.
Looking up the t-distribution table with 48 degrees of freedom, we find that the area beyond -2.00 is 0.0257, and the area beyond 2.00 is also 0.0257. So the p-value is:
p-value = 0.0257 + 0.0257
p-value = 0.0514
Rounding to three decimal places, the p-value of the test is 0.051.
Therefore, at a significance level of 0.05, we fail to reject the null hypothesis and conclude that we do not have sufficient evidence to support the claim that the mean GPA for Psychology students at the college is equal to 3.2.
Learn more about Hypothesis visit,
brainly.com/question/11555274
#SPJ4
Use the reciprocal property to solve for y: 15/y = 20/10
Answers
Answer:
15/2
Step-by-step explanation:
15/y = 20/10
Cross multiply
150=20y
y=15/2
The value of y will be;
⇒ y = 7.5
What is Multiplication?
To multiply means to add a number to itself a particular number of times. Multiplication can be viewed as a process of repeated addition.
Given that;
The expression is,
⇒ 15 / y = 20 / 10
Now,
Solve the expression for y by using reciprocal property as;
The expression is,
⇒ 15 / y = 20 / 10
Apply reciprocal property;
⇒ y / 15 = 10 / 20
⇒ y = 15 × 10/20
⇒ y = 15 × 1/2
⇒ y = 7.5
Thus, The value of y will be;
⇒ y = 7.5
Learn more about the reciprocal visit:
https://brainly.com/question/15791951
#SPJ2
Reduce to a simplest form.
1. 6/20
2. 28/16
Answers
Answer:
D
Step-by-step explanation:
2.) 7/4 or 1 3/4 i reduced the fraction by dividing it by 2 then 2 again and for the other one i divided 16 into 28 and made it into a mixed number then reduced
14. If I share 123 sweets equally among amongst 7 children, how many sweets will each child get?
……………………………………..
Answers
Answer:
No. of sweets = 123
No. of children = 7
then
sweets per children =123/7 =17. 57
Answer: =17.57
Step-by-step explanation:
Divide the no. of sweets by the no. of children
=123/7
=17.57
y = |x-5| + |x+5| if x<-5
Answers
Hence, Y = -2x, For x < -5, The Function Y = | x - 5 | + | x + 5 |
Therefore, For: x < -5, The Function: Y = | x - 5 | + | x + 5 |
Simplifies to: Y = -2x
Step-by-step explanation:ANALYSIS:To solve the problem, We need to consider the definition of the ABSOLUTE VALUE FUNCTION and the given condition: x < -5
We will break the absolute value expressions into their respective cases and simplify the equation.
BREAK THE ABSOLUTE VALUE EXPRESSIONS:Since: x < -5, We now have:
x - 5 < 0 and x + 5 < 0
So, The absolute value expressions can be written as:| x - 5 | = -(x - 5) and | x + 5 | = -(x + 5)
Now, we substitute these expressions into the equation:Y = -(x - 5) + ( -(x + 5))
Simplify:Y = -x + 5 - x - 5
Combine Like Terms:Y = -2x
Draw the conclusion:For x < -5, The Function Y = | x - 5 | + | x + 5 |
Simplifies to: Y = -2x
Hence, Y = -2x, For x < -5, The Function Y = | x - 5 | + | x + 5 |
I hope this helps you!
What problems came from the borders drawn by Great Britain and France in Southwest Asia?
Answers
The borders drawn by Great Britain and France in Southwest Asia (specifically during the period of the Sykes-Picot Agreement and the subsequent mandates) have had various consequences and problems. Here are some of the key issues that arose:
Arbitrary divisions: The borders created by these colonial powers often disregarded existing ethnic, religious, and tribal boundaries.
Creation of unstable states: The borders established by Britain and France created new nation-states, such as Iraq, Syria, Lebanon, Jordan, and Palestine, without taking into account the underlying political, ethnic, and religious dynamics.
Geopolitical rivalries: The borders created by colonial powers also served their geopolitical interests rather than the aspirations and needs of the local populations.
Thus, these problems came from the borders drawn by Great Britain and France in Southwest Asia.
For more details regarding Sykes-Picot Agreement, visit:
https://brainly.com/question/32052226
#SPJ1
The formula for area of a trapezoid is A =1/2 h(b1 + b2). Express h in terms of A, b1 and b2
Answers
Answer:
H is the height
Step-by-step explanation:
A= Area
B= Base
1/2= 0.5
Find the value of x
.

Answers
Answer:
x = 9
Step-by-step explanation:
5x + 27 = 45 + 3x
Subtract 3x from both sides
2x + 27 = 45
Subtract 27 from both sides
2x = 18
Divie both sides by 2
x = 9
The approximate length of side XY is a: 3b: 3.16c: 4d: 4.24 units.

Answers
Solution
Given a triangle XYZ on the graph
The coordinates of points X, Y and Z are
\(\begin{gathered} X\Rightarrow(-3,-1) \\ Y\Rightarrow(-2,-4) \\ Z\Rightarrow(-6,-4) \end{gathered}\)To find the approximate length of the sides, we apply the formula to find the distance, d, between two points which is
\(d=\sqrt{(x_2-x_2)^2+(y_2-y_1)^2}\)To find the approximate length of side XY, substitute the coordinates of points X and Y into the formula to find the distance, d, between two points
\(\begin{gathered} XY=\sqrt{(-3-(-2))^2+(-4-(-1))^2} \\ XY=\sqrt{(-1)^2+(-3)^2} \\ XY=\sqrt{1+9}=\sqrt{10}=3.16\text{ units} \\ XY=3.16\text{ units} \end{gathered}\)Hence, the approximate length of side XY is 3.16 units (option b)
a penny is dropped from a height of 144 ft. Calculate the time between when the rock was dropped and when it landed. If we choose "down" as positive and ignore air friction, the function is h(t) = 16t^2 - 144.
answers
t = 9seconds
t = 3 seconds
t = 18 seconds
t = 10 seconds
Answers
Answer:
The time of fall is 9 seconds
Step-by-step explanation:
We are asked to find the time between when the penny was dropped and when it landed.
To do this, we have to start with what we are given and see how we can relate it to find what we are looking for.
From the problem, we are given the equation which represents the height which the penny travels as it falls. It is given by
\(h(t) = 16t^2 - 144.\) ----------- equation 1
in addition to that, we are also given that h = 144ft at the end of the fall; that is, where t = t max, and h = hmax
Hence, it is safe to say that at the end of the fall, that the heigh is no more changing. This means that dh/dt = 0
We can now differentiate equation 1 above to get
h max = 16t - 0 ------------- equation 2
recall that h max = 144
plugging the value of h max into equation 2, we have
144 = 16t
t = 9 seconds
Therefore the time of fall is 9 seconds
Answer:
t= 3 seconds
Step-by-step explanation:
I took the quiz and this is the answer
Find th percent in the fastest way. 6 1/4% of $3872
Answers
keeping in mind that 6¼ is just 6 + ¼ or 6 + 0.25 or just 6.25
\(\begin{array}{|c|ll} \cline{1-1} \textit{a\% of b}\\ \cline{1-1} \\ \left( \cfrac{a}{100} \right)\cdot b \\\\ \cline{1-1} \end{array}~\hspace{5em}\stackrel{\textit{6.25\% of 3872}}{\left( \cfrac{6.25}{100} \right)3872}\implies 242\)
Solve for x:
5x−29>−34 OR 2x+31<29
A. x<−1 OR x>−1
B. x<−1
C. There are no solutions
D. All values of X are solutions
Answers
The solution for the inequalities is x > - 1 OR x < -1.
What is linear inequality?The mathematical expression for inequality states that neither side is equal. In mathematics, inequality occurs when the relationship results in a non-equal comparison between two expressions or numbers. Any of the inequality symbols, such as greater than (>), less than (<), greater than or equal to (≥), less than or equal to (≤), or not equal to (≠).
Given : 5x - 29 > -34 OR 2x + 31 < 29.
to solve the equations,
5x - 29 > -34 OR 2x + 31 < 29.
For 5x - 29 > -34
On adding both sides by 29.
5x > - 34 + 29 .
5x > -5
On dividing both sides by 5.
x > - 1 .
For 2x + 31 < 29.
On subtracting both sides by 31
2x < 29 -31
2x < - 2
On dividing both sides by 2.
x < -1
So , x > - 1 OR x < -1 .
Therefore, option A is correct.
Learn more about linear inequality;
https://brainly.com/question/11897796
#SPJ1
(q7) Find the volume of the solid obtained by rotating the region bounded by y = x and y = 2x2 about the line y = 2.

Answers
The volume of the solid obtained by rotating the region bounded by y = x and y = 2x² about the line y = 2 is π/24 units cube.
option D.
What is the volume of the solid obtained?The volume of the solid obtained by rotating the region bounded by y = x and y = 2x² about the line y = 2 is calculated as follows;
y = 2x²
x² = y/2
x = √(y/2) ----- (1)
x = y -------- (2)
Solve (1) and (2) to obtain the limit of the integration.
y = √(y/2)
y² = y/2
y = 1/2 or 0
The volume obtained by the rotation is calculated as follows;
V = 2π∫(R² - r²)
V = 2π ∫[(√(y/2)² - (y)² ] dy
V = 2π ∫ [ y/2 - y² ] dy
V = 2π [ y²/4 - y³/3 ]
Substitute the limit of the integration as follows;
y = 1/2 to 0
V = 2π [ 1/16 - 1/24 ]
V = 2π [1/48]
V = π/24 units cube
Learn more about volume of solid here: https://brainly.com/question/24259805
#SPJ1
26(917
What is the answer with remainder?
Answers
Answer: It has a remainder of 26, hope this helps <3
Two candidates were running for mayor. Luke earned more than half of the popular vote. Which of the following could be the percentage of votes he won?
A:34%
B:65%
C:22%
D:49%
Answers
Answer:
65% because 65 is more than half..And half is 50, 65 is more than 50
whats 80xa=7200xb=51840000
Answers
If [(80 * a) = (7200 * b) = 51840000], then solving this equation, we get the values of the variables "a" as 648000, and "b" as 7200.
As per the question statement, we are provided with an equation containing two variables, "a" and "b" which is
[(80 * a) = (7200 * b) = 51840000].
We are required to calculate the values of "a" and "b" from the above mentioned equation.
Let us consider the part [(80 * a) = 51840000] of our concerned equation, solving which, we get,
[(80 * a) = 51840000]
Or, [a = (51840000/80)]
Or, (a = 648000)
Similarly considering the part [(7200 * b) = 51840000] of our concerned equation, solving which, we get,
[(7200 * b) = 51840000]
Or, [b = (51840000/7200)]
Or, (b = 7200)
Equation: An equation is a mathematical statement that determines the relation of equality among two expressions, by a connector "equal to" sign in between. Variable: In mathematics, a variable is an alphabet used as a symbol and placeholder for any mathematical object, particularly to represent a number, a vector, a matrix, a function, the argument of a function, a set, or an element of a set.To learn more about Equations and Variables, click on the link below.
https://brainly.com/question/22277991
#SPJ1
the assembly time for a product is uniformly distributed between 6 and 10 minutes. the standard deviation of assembly time (in minutes) is approximately . a. .33 b. .13 c. 16 d. 1.15
Answers
The standard deviation of assembly time (in minutes) is approximately .33.
The standard deviation of a uniform distribution of assembly time is calculated using the following formula:
σ = √((b-a)^2 / 12),
where a is the lower boundary of the range of assembly time (6 minutes) and b is the upper boundary of the range of assembly time (10 minutes).
Substituting a = 6 and b = 10 into the formula yields:
σ = √((10-6)^2 / 12)
σ = √(16/12)
σ = √(1.33)
σ = .33
Therefore, the standard deviation of assembly time (in minutes) is approximately .33.
Learn more about standard deviation here:
https://brainly.com/question/23907081
#SPJ4
air is pumped into a spherical balloon at the constant rate of 200 cubic centimeters per second. how fast is the surface area of the balloon changing when the radius is 5 centimeters?
Answers
The rate of change of the surface area of the balloon is 32cm²/sec
We know that volume of the sphere is,
V = 4/3πr³
Given,
dV / dt = +200
r = 5cm
d(SA) / dt = ?
dV / dt = 4πr² dr/dt
80 = 4π (25) dr/dt
dr / dt = 0.8 / π
SA = 4πr²
d(SA) / dt = 8πr dr / dt
d(SA) / dt = 8π × 5 × 0.8 / π
d(SA) / dt = 32cm²/sec
Hence the rate of change of the surface area is 32cm²/sec
To learn more about differential equations,
https://brainly.com/question/14620493
#SPJ4
PLEASE help stuck on this one and need helpppp ill mark you brainliest nmw

Answers
The images of the coordinates of the quadrilateral are A'(x, y) = (0, 0), B'(x, y) = (2, - 5), C'(x, y) = (- 5, - 5) and D'(x, y) = (- 3, 0). (Correct choice: B)
How to determine the image of a set of points by rotation
In this problem we have the coordinates of the four ends of a quadrilateral, whose images must be found by rotation formula:
P'(x, y) = (x · cos θ - y · sin θ, x · sin θ + y · cos θ)
Where:
x, y - Coordinates of the original point.θ - Rotation angle, in degrees.If we know that A(x, y) = (0, 0), B(x, y) = (5, 2), C(x, y) = (5, - 5), D(x, y) = (0, - 3) and θ = 270°, then the coordinates of the images are, respectively:
A'(x, y) = (0 · cos 270° - 0 · sin 270°, 0 · sin 270° + 0 · cos 270°)
A'(x, y) = (0, 0)
B'(x, y) = (5 · cos 270° - 2 · sin 270°, 5 · sin 270° + 2 · cos 270°)
B'(x, y) = (2, - 5)
C'(x, y) = (5 · cos 270° + 5 · sin 270°, 5 · sin 270° - 5 · cos 270°)
C'(x, y) = (- 5, - 5)
D'(x, y) = (0 · cos 270° + 3 · sin 270°, 0 · sin 270° - 3 · cos 270°)
D'(x, y) = (- 3, 0)
To learn more on rotations: https://brainly.com/question/1571997
#SPJ1
the vertices of the trapezoid are the origin along with a (4m, 4n), b (4q, 4n), and c (4p, 0). find the midpoint of the midsegment of the trapezoid.
Answers
The midpoint of the midsegment of Trapezoid is E(2m, 2n) and F (2(q+p), 2n).
According to the question,
The vertices of the trapezoid are A(4m , 4n) , B(4q , 4n) , C(4p , 0) and D(0,0).
Let mid-point of midsegment of Trapezoid be E and F
Using section formula
E (x- coordinate)= 1*4m + 0 /2
E (x- coordinate)=2m
E (Y- coordinate)= 4n/2
E (Y- coordinate)=2n
So, the coordinates of E (2m, 2n)
similarly,
F (x- coordinate)= (1*4q + 1*4p)/2
F (x- coordinate)=2(p + q)
F (Y- coordinate)= 4n/2
F(Y- coordinate)=2n
So, the coordinates of F (2(q +p), 2n)
Learn more about this Trapezoid here:
https://brainly.com/question/11908553
#SPJ4
please help its due today


Answers
The measure of the angles are: K, L, C, N, O G = -3/4, 65, 8 40.4, 89 and 44 respectively
How to find the measure of the angles?To determine K
9x + 2 = 23 + 5x -1
9x -5x = -1 +2
4x = -3Making x the subject we have
x= -3/4
To find the value of L
Let angle L be x
x + 65 = 130
x=130-65
That is x = 65
To find the m<C
4x + 7 = 3x + 15
Collecting like terms
4x - 3x = 15-7
Making x the subject of the relation we have
x=8
To find the m<N
7x + 6x - 1 = 90
13x = 89
x=89/13
x= 6.9
Substitute x = 6.9 in 6x -1
6(6.9) -1
m<L = 40.4
To find the m<O
5x - 11 = 4x +9
5x-4x = 9 +11
x=20
By substitution in 4x +9 we have
4(20) +9
80+9
m<S = 89
To find the value of the angle G
6x - 4 = 5x +4
6x - 5x = 4+4
x = 8
Therefore the m<G is
5x +4
5(8) +4
40+4 = 44 digress
Learn more about measure of angles in https://brainly.com/question/28451077
#SPJ1
x^2−19x+c Find the value of c that makes the trinomial a perfect square.
Answers
The value of c that makes the trinomial a perfect square is 180.25.
Trinomial perfect squares:A trinomial perfect square is a trinomial expression that can be written in the form: (a + b)² = a²+ 2ab + b² Where a and b are constants.
To determine if a trinomial is a perfect square, we can check if it can be written in this form.
Let's take a perfect square (x - k)² where k is some constant.
Expanding (x - k)², we get:
x² - 2kx + k² ---- (1)
Compare (1) with x² −19x + c
=> -2k = -19
=> k² = c
From the first equation, we can solve for k:
k = 19/2
Substituting this into the second equation, we can solve for c:
c = k² = (19/2)² = 90.25
Therefore,
The value of c that makes the trinomial a perfect square is 180.25.
Learn more about Trinomial perfect squares at
https://brainly.com/question/16615974
#SPJ1
the following are the ages in years of members of group 8, 11, 8,10 ,6, 7, 3x ,11, 11
if the mean age is 9years
find:
1.x
2.find the modal age
3.find the median
Answers
Answer:
X = 3
Mode: 11median: 9
Step-by-step explanations
3x = 3*2 = 6
(6,7,8,8,9,10,11,11,11)/9 = 81/9 = 9
The value of X = 3
Mode: 11
Median: 9
What are mean and median?The mean is the average value which can be calculated by dividing the sum of observations by the number of observations
Mean = Sum of observations/the number of observations
Median represents the middle value of the given data when arranged in a particular order.
Mode is that number that appears most frequently in the given set of data.
We are given that the ages in years of members of group 8, 11, 8,10 ,6, 7, 3x ,11, 11 if the mean age is 9 years
3x = 3(2 )= 6
Mean = (6,7,8,8,9,10,11,11,11)/9
= 81/9
= 9
Learn more about mean and median;
https://brainly.com/question/17060266
#SPJ2
Help me pleaseeeeee!!!

Answers
♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️
\( \tan(x) = \frac{12}{29} \\ \)
\( \tan(x) = 0.413\)
\(x≈ 22.475\)
\(x ≈22.5\)
♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️